Bending Verification Of Reinforced Concrete {Eurocode}

Bending is the verification I do the most when I design reinforced concrete elements as a structural engineer.

You need to verify bending when you design beams, slabs, frames, foundation beams, pad and strip footings and many more structural elements.

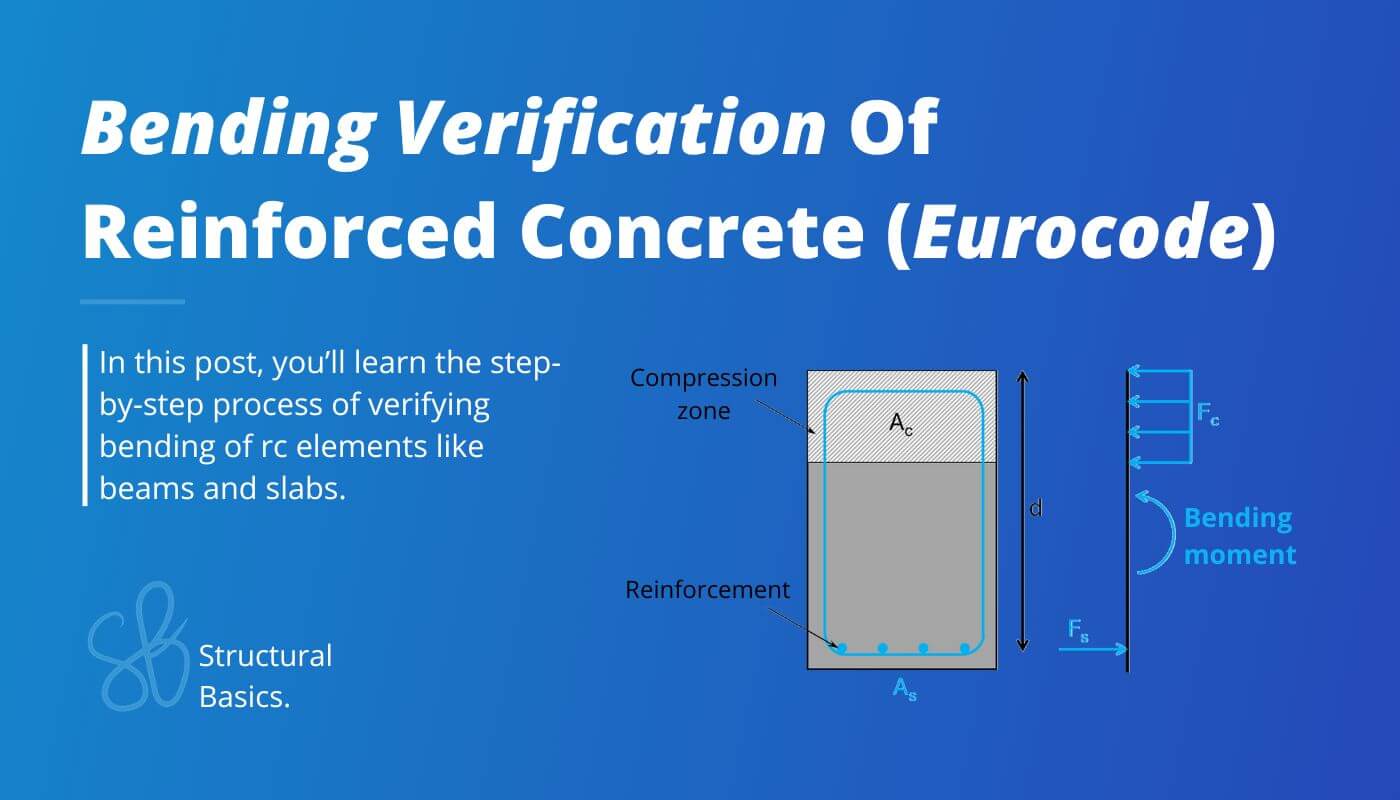
Concrete is great in compression but very weak in tension. The steel reinforcement is added to take up the tension forces. The bending moment of a rc cross-section is split up in a compression and a tension component, where the compression component is a compression zone taken up by the concrete and the tension force is taken up by the reinforcement as a tension force.

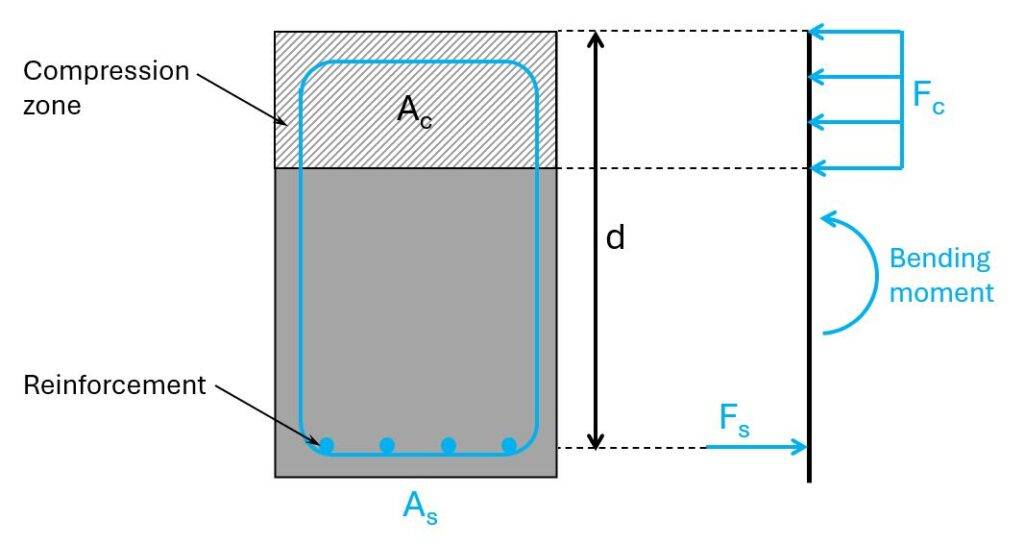
In this article, I’ll show how you design and verify reinforced concrete elements like beams and slabs for a bending moment in 6 steps.

Let’s get into it.

6 Steps To Verify Bending Of Reinforced Concrete

To run through the #6 steps to verify bending, we’ll use the following beam cross-section. You can apply this workflow also for slabs. Instead of the width of the beam in the formulas, use 1m.

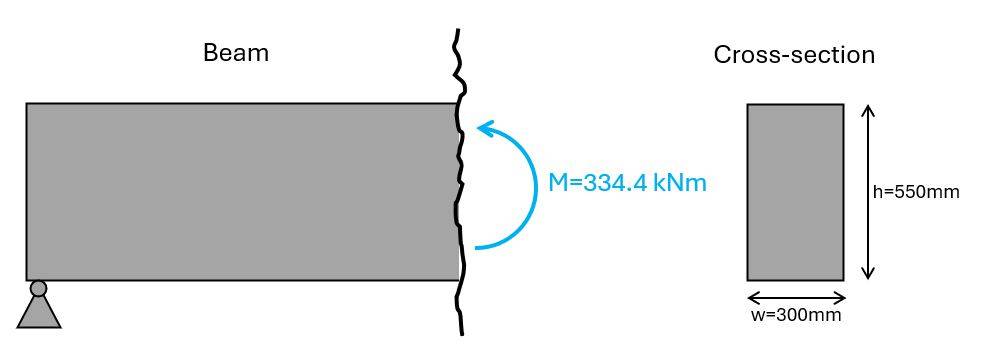
In the literature, you can find many different formulas for the bending verification. The following formulas are the ones I use. Feel free to use different formulas if you want. Just know that there are options and the one I am presenting is not the one-and-only.

Also, every engineer has its own workflow. Some will first calculate the minimum reinforcement and other pick the reinforcement before they calculate the required reinforcement.

Therefore, feel free to swap around the following steps.

View this post on Instagram

A post shared by Structural Basics | Structural Engineering (@structural.basics)

Step #1: Define the properties of concrete and reinforcement

In this tutorial, we’ll use the following concrete and reinforcement properties.

Concrete compression strength	fc.k = 25 MPa
Concrete tensile strength	fctm = 2.6 MPa
Partial factor in-situ concrete (Denmark)	γc = 1.45
Design compression strength	fc.d = fc.k/γc = 17.2 MPa
Design tensile strength	ft.d = fctm/γc = 1.8 MPa
Concrete cover	c = 30 mm
Reinforcement yield strength	fy.k = 550 MPa
Partial factor for reinforcement (Denmark)	γs = 1.2
Design yield strength	fy.d = fy.k/γs = 458.3 MPa
For concrete C12-C50	εcu3 = 0.35
For concrete C12-C50	εc3 = 0.175
For concrete C12-C50	λ = 0.8
Reinforcement 550 MPa	εy.d = 0.208
E-modulus concrete	Ecm = 31476 MPa
E-modulus reinforcement	Es = 200000 MPa

Step #2: Calculate the lever arm of the longitudinal reinforcement

The lever arm is the distance from the most outer concrete fibre in compression to the longitudinal reinforcement taking up the tension forces.

In our example (for a positive bending moment), we’ll get compression in the top and tension in the bottom.

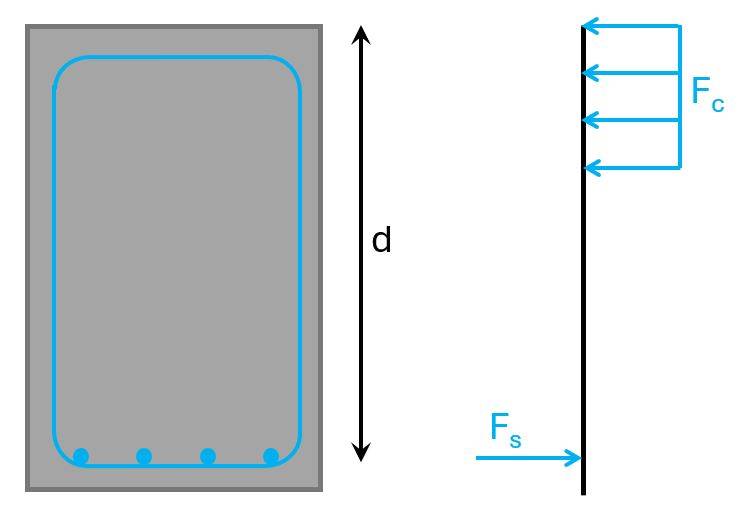
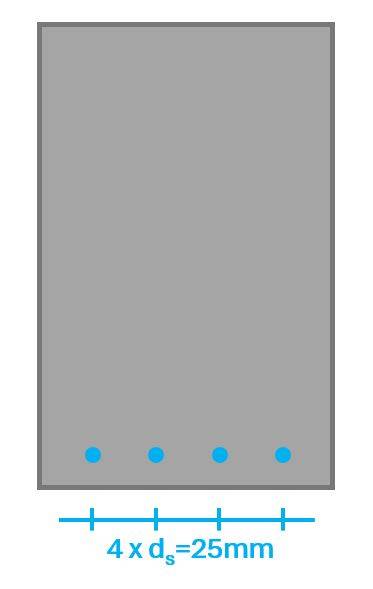
Let’s assume the diameter of the longitudinal reinforcement is d_s = 25 mm and the stirrups d_v = 10 mm, then the lever arm is calculated as:

Step #3: Calculate the required longitudinal reinforcement to take up the tension force

The required reinforcement is calculated with the following formulas.

Required longitudinal reinforcement:

Step #4: Pick the diameter and amount of longitudinal reinforcement

We picked a rebar diameter of 25 mm, which has a cross-sectional area of:

Next, we calculate the amount of rebars we need as a minimum to fulfill the required reinforcement demand:

Now, we can calculate the total reinforcement area for 4 rebars of diameter 25 mm.

Step #5: Verify the degree of reinforcement

Now, we basically verify that the cross-section is not over and underreinforced.

Verification:

Verification:

Verification:

Step #6: Verify the minimum reinforcement

The minimum reinforcement is calculated with EN 1992-1-1 9.2.1.1 (9.1N):

Now, comparing A_s with A_s.min shows that the minimum reinforcement is less than the reinforcement we get from 4 x d=25mm rebars.

Now, this was only the verification for the bottom longitudinal reinforcement. Don’t forget to also verify the reinforcement in the top. If you don’t have negative moments, it should at least have the minimum reinforcement.

Final words

This is how you verify bending for reinforced concrete.

For many of you, this might have been repetition. But repetition is always good. It deepens your knowledge.

I also often gain new insights when I write and document these newsletters. Even though I have probably done this verification many times.

What happens in your day-to-day tasks as a structural engineer is that you use software for most verifications. So you actually don’t go through each step, we went through. But you definitely need to know what the software is doing.

Going through these hand calculations helps remember the formulas and fundamentals of reinforced concrete design. If we know the fundamentals by heart, we have a much better understanding of why a structure might not verify in a software program and how we can make it verify.

If you don’t want to miss any new structural design tutorials, then subscribe to our free weekly newsletter.